Merge Sort Algorithm - The Coding Shala

Last Updated: 20-Jan-2021

Home >> Algorithms >> Merge Sort Algorithm

 In this post, we will learn what is Merge Sort Algorithm and how it works. We will write a Java Program for Merge Sort.

Merge Sort Algorithm

Merge Sort is an efficient and general-purpose sorting algorithm. It is a classic example of the divide-and-conquer algorithm.

The merge sort algorithm can be divided into three steps, like all the divide-and-conquer algorithm:

step 1. Divide the given unsorted list into several sublists. (Divide)

step 2. Sort each of the sublists recursively. (Conquer)

Step 3. Merge the sorted sublists to produce a new sorted list. (Combine)

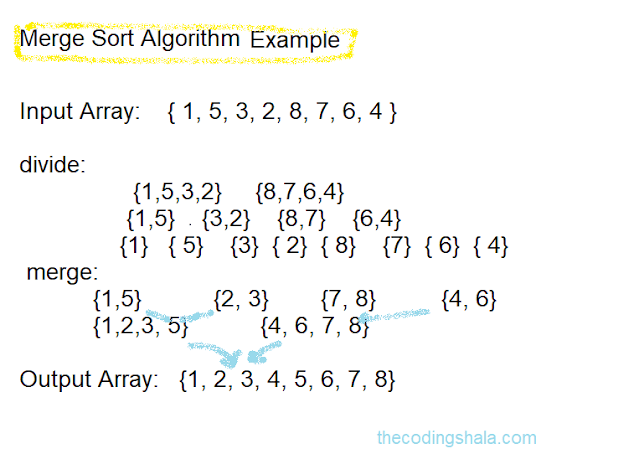
Here is an example that explains the working of the merge sort algorithm:

The time complexity of Merge Sort Algorithm

The time complexity of the merge sort algorithm is O(NlogN), where N is the length of the input list.

Merge Sort Algorithm Java Program

Java Program: 

import java.util.Arrays;

//merger sort
//the coding shala

class Main{
	
	public static int[] merge_sort(int[] input) {
		int len = input.length;
		if(len<=1) return input;
		
		int mid = len/2; 
		//divide into left and right array by mid
		int[] left = merge_sort(Arrays.copyOfRange(input, 0, mid));
		int[] right = merge_sort(Arrays.copyOfRange(input, mid, len));
		
		//merge both arrays
		return merge(left, right);
	}
	
	public static int[] merge(int[] left, int[] right) {
		int len_left = left.length;
		int len_right = right.length;
		int[] result = new int[len_left+len_right];
		
		int left_index = 0, right_index = 0, result_index = 0;
		//combine the arrays in order
		while(left_index < len_left && right_index < len_right) {
			if(left[left_index] < right[right_index]) {
				result[result_index] = left[left_index];
				result_index++;
				left_index++;
			}else {
				result[result_index] = right[right_index];
				result_index++;
				right_index++;
			}
		}
		
		//if left array elements remains
		while(left_index < len_left) {
			result[result_index] = left[left_index];
			result_index++;
			left_index++;
		}
		
		//if right array element remains
		while(right_index < len_right) {
			result[result_index] = right[right_index];
			result_index++;
			right_index++;
		}
		
		return result;
	}
	
	public static void main(String[] args) {
		int[] input = {1,5,3,2,8,7,6,4};
		input = merge_sort(input);
		for(int i=0;i<input.length;i++) {
			System.out.print(input[i]+" ");
		}
	}
}

Other Posts You May Like

• Insertion Sort Algorithm
• Counting Sort Algorithm
• Bucket Sort Algorithm
• Bubble Sort Algorithm
• Binary Search Algorithm

Please leave a comment below if you like this post or found some errors, it will help me to improve my content.